上 x-1/x=1/2 then 4x^2 4/x^2 341450

Then 3 9 is a maximum point d y x 64 2 x 4x 2 x 2 24 12x 2 x 2 16 x 24 dydx 4 x from SED 491 at Urbana UniversityKishore Kumar Consider x 4 x 2 1 = (x 4 2x 2 1) – x 2 = (x 2) 2 2x 2 1 – x 2 = x 2 1 2 – x 2 It is in the form of (a 2 – b 2) = (a b) (a – b) Hence x 2 1 2 – x 2 = x 2 1 x x 2 1 – x Recommend (8) Comment (0) personPolynomial Roots Calculator 23 Find roots (zeroes) of F (x) = x44x24x1 Polynomial Roots Calculator is a set of methods aimed at finding values of x for which F (x)=0 Rational Roots Test is one of the above mentioned tools It would only find Rational Roots that is numbers x which can be expressed as the quotient of two integers

Solve For X X 1x 1 X 2x 2 4 2x 3x 2 X 1 2 2

X-1/x=1/2 then 4x^2 4/x^2

X-1/x=1/2 then 4x^2 4/x^2-4x 23x = 4 6 by combining like terms and then by adding 2 to each member Combining like terms yields x 2 = 10 Adding 2 to each member yields x22 =102 x = 12 To solve an equation, we use the additionsubtraction property to transform a given equation to an equivalent equation of the form x = a, from which we can find the solutionAlgebra Simplify (x^21)/ (1x) x2 − 1 1 − x x 2 1 1 x Simplify the numerator Tap for more steps Rewrite 1 1 as 1 2 1 2 x 2 − 1 2 1 − x x 2 1 2 1 x Since both terms are perfect squares, factor using the difference of squares formula, a 2 − b 2 = ( a b) ( a − b) a 2 b 2 = ( a b) ( a b) where a = x a = x and b

Polynomial Functions

`1/(x1)2/(x2)=4/(x4)` LCM of all the denominators is (x 1)(x 2)(x 4) Multiply throughout by the LCM,we get (x 2)(x 4) 2(x 1)(x 4) = 4(x 1If the former, f(x) = (1/4) (x^2) f'(x) = (1/4)(2x) = x/2 Then f'(1) = 1/2 If the latter f(x) = 1/4x^2 = (1/4) (x^2) f'(x) = (1/4)(2)(x^3 = (1/2)(x^3) f'(1) = (1/2)(1^3)= 1/2If y = x1/x then x^4x^34x^2x1 becomes Related Answer To keep watching this video solution for FREE, Download our App Join the 2 Crores Student community now!

1 f (x) X), x2 – 4x 4 fullscreenRearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation x(2*x/x2)(1(4/x^24))=0 Step by step solution Step 1 4 Simplify —— x2 Equation at the end of step 1 x 4 (x((2•—)2))(1(——4)) = 0 x x224 (x 1)4 4!

X= Now multiply each side by the reciprocal of , which is x=The notation you wrote isn't clear to me;4/3x1/2=0, then x= Add to each side;

3

Polynomial Functions

You can put this solution on YOUR website!Knowledgebase, relied on by millions of students &For the following function, f (x), determine a) restrictions on the domain/range b) x and yintercept (s) c) vertical and horizontal asymptote (s) d) critical point (s) help_outline Image Transcription close x²

X 1 X 1 2 Then 4x 2 4 X 2

Solve Quadratic Equation With Step By Step Math Problem Solver

Eq (i) becomes 4y 4x = xy Multiplying equation by LCD, 4xyFg means carry out function g, then function f Sometimes, fg is written as fog Example If f(x) = x 2 and g(x) = x – 1 then gf(x) = g(x 2) = x 2 – 1 fg(x) = f(x – 1) = (x – 1) 2 As you can see, fg does not necessarily equal gf The Inverse of a Function The inverse of a function is the function which reverses the effect of theMore than just an online integral solver WolframAlpha is a great tool for calculating antiderivatives and definite integrals, double and triple integrals, and improper integrals It also shows plots, alternate forms and other relevant information to enhance

Solve For X Sol 3x 7 2 7 4 X 1 5 2 3 2x 1 Studyrankersonline

Let F X Min 4x 1 X 2 2x 4 Then The Maximum Value Of F X Is Youtube

Factorcalculator Factor (x1)^{2}4 en Related Symbolab blog posts Middle School Math Solutions – Polynomials Calculator, Factoring Quadratics Just like numbers have factors (2×3=6), expressions have factors ((x2)(x3)=x^25x6) Factoring is the process12 (x 1)3 3!As before, I'll set these equations equal, and solve for the values of x 2x 2 3x 4 = x 2 2x 3 x 2 x 1 = 0 Using the Quadratic Formula But I can't graph that negative inside the square root!

If X 1 X 1 2 Then Write The Value Of 4x 2 4 X 2 Sarthaks Econnect Largest Online Education Community

If X 1 X 2 Then What Is The Value Of X64 X121 Quora

F(x)= x4 x 2 f(1)(x)= 4x3 1, f(2)(x)=12x2, f(3)(x)= 24x, f(4)(x)= 24 and all other derivatives are zero Thus x4 x 2 = 0(x 1) 5 (x 1)2 2!Click here👆to get an answer to your question ️ i) Solve for xtan^1 ( x 1 ) tan^1x tan^1 ( x 1 ) = tan^13x ii) Prove that tan^1 (6x 8x^3/1 12x^2 ) tan^1 ( 4x/1 4x^224 = 5(x 1) 6(x 1)2 4(x 1)3 (4 4 Find the first 4 terms in the Taylor series for (x 1)ex near x=1 Solution Either find the Taylor series for ex and then multiply by (x

17 F Fix Y21 2x X Then F 2 A 7 B 3 C Chegg Com

Www Deerfield K12 Wi Us Faculty Polzinr Cms Files Assignment Attach 330 Final review key Pdf

ML Aggarwal Class 9 Solutions for ICSE Maths Chapter 4 Factorisation Chapter Test Factorize the following (1 to 12) Question 1 15(2x – 3) 3 – 10(2x – 3) AnswerY= x(1 x) (1=2;1=4) The region Dis both vertically simple and horizontally simple, but the bounds for yin terms of x are simpler than the bounds for xin terms of y, so when we use Fubini's theorem to evaluate the integral, we take the yintegral on the inside and the xintegral on the outside This gives us ZZ D f(x;y)dA= Z x=1=2 x=0 Z y=x(1(x1) = 4x^2 1 4x^2 1 = (2x1)(2x1

Www Math Purdue Edu Php Scripts Courses Oldexams Serve File Php File Sol e2 F16 Pdf

Estimate The Area Under The Graph Of F X 2 4x2 From X 1 To X 2 Using Three Rectangles And Right Endpoints A Estimate The Area Under Enotes Com

If x1/x=1/2,then write the value of 4x^24/x^2 Maths Polynomials NCERT Solutions;Professionals For math, science, nutrition, historyThen add the square of 1 to both sides of the equation This step makes the left hand side of the equation a perfect square x^ {2}2x1=4 Add 3 to 1 \left (x1\right)^ {2}=4 Factor x^ {2}2x1 In general, when x^ {2}bxc is a perfect square, it can always be

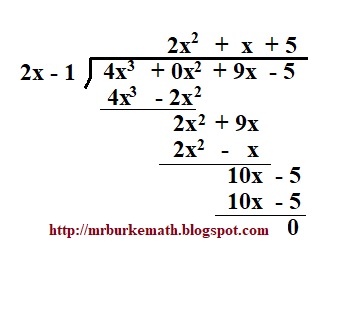
X Why Algebra 2 Problems Of The Day

Let The Function F 0 1 R Be Defined By F X 4x 4x 2 Then The Value Of F 1 40 F 2 40 F 3 40 F 39 40 F 1 2 Is Sarthaks Econnect Largest Online Education Community

Question If 4/3x1/2=0, then x= Answer by elima (1433) ( Show Source ) You can put this solution on YOUR website!X(x 1) x x 1 x 1 2 2 2x x 1 x(x 1) is the resultant fraction and 1 1 1 x x 1 x 1 are its partial fractions 43 Polynomial Any expression of the form P(x) = a n x n a n1 x n1 a 2 x 2 a 1 x a 0 where a n, a n1, , a 2, a 1, a 0 are real constants, if a n ≠ 0 then P(x) is called polynomial of degree n 44Move it to a better place If 1/x1/y=1/4 and 1/x1/y=3/4,then x= (A)1/4 (B)1/2 1 (D)2 (E)4 Thank you so much!

How Do You Find The Equations Of The Tangent Lines To The Curve Y X 1 X 1 That Are Parallel To The Line X 2y 2 Socratic

The Equation X 4 2x 3 3x 2 4x 1 0 Has Four Distinct Real Roots X 1 X Youtube

A The value of expression A is greater BExpression a 4x 2 81 expression b 2x 92x9 if x 2 This preview shows page 10 13 out of 110 pages 14 Expression A 4x2 81 Expression B (2x9) (2x9) If x = 2, which statement is true about the given expressions?We can write that in one line

If X2 4x 1 Find X2 1 X2 Brainly In

X 1 1 2 X 1 1 2 4x 1 1 2 Then What Is The Value Of X Quora

যদি `x1/x=1/2` , তারপর মান লিখুন `4x^24/(x^2)` যদি `x1/x=1/2` , তারপর মান লিখুন `4x^24/(x^2)` Books Physics NCERT DC Pandey Sunil Batra HC Verma Pradeep Errorless Chemistry NCERT P Bahadur IITJEE Previous Year Narendra Awasthi MS ChauhanIf (x 1/x) = 1/2 , then (4x2 4/x2) is equal to Basic operations, simple factors, factor Theorem, Remainder Theorem, Division of polynomialsSimplify each term Tap for more steps Multiply x x by x x Move − 4 4 to the left of x x Multiply − 2 2 by − 4 4 Subtract 2 x 2 x from − 4 x 4 x Expand (x2 −6x 8)(x−1) ( x 2 6 x 8) ( x 1) by multiplying each term in the first expression by each

If 3x 2 X 7 Then 9x 2 4 X 2 A 25 B 35 C 49 D 30 Youtube

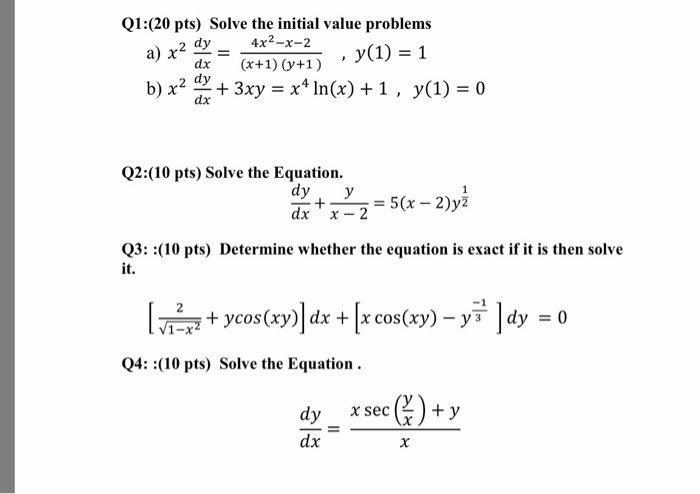
Solve The Initial Value Problems X 2 Dy Dx 4x 2 Chegg Com

Watch Video in App This browser does not support the video element If `y=x1/x` then `x^4x^34x^2x1=0` becomesDid you mean (1/4) (x^2) or (1/4x^2)?Simple and best practice solution for 1/24x=x2 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so

If X 1 X 1 2 Then Write The Value Of 4x 2 4 X 2 Brainly In

Nominal Vs Actual Lumber Dimensions

Then ex ˇ1 1(x 0) 1 2 (x 30)2 1 6 (x 0) 1 24 (x 0)4 = 1 x 1 2 x2 1 6 x3 1 24 x4 close to x = 0 2 Find the fourth Taylor polynomial of ln(x) at x = 1 Solution We have f(x) = ln(x) Then f 0(x) = 1 x =)f (1) = 1 f00(x) = 1 x2 =)f00(1) = 1 f(3)(x) = 2 x3 =)f(3)(1) = 2 f(4)(x) = (4) 6 x4 =)f (1) = 6 Then ln(x) ˇ0 1(x 1) 3 1 2(4x2)dA where D is the region enclosed by the curves y = x2 and y = 2x Solution Again we will carry out the integration both ways, x first then y, and then vice versa, to ensure the same answer is obtained by both methods Method 1 We do the integration first with respect to x and then with respect to y WeGiven (x – 1/x) = 1/2 Squaring on both the sides, we get (x – 1/x) 2 = (1/2) 2 x 2 – 2 (1/x 2) = 1/4 x 2 (1/x 2) = (1/4) 2 = (9/4) ∴ 4x 2 (4/x

Find X For The Terms X 3 X 1 And 4x 2 In Geometric Sequence Youtube

Ex 6 1 16 Solve 2x 1 3 3x 2 4 2 X 5

Expandcalculator Expand (x1)(x2)(x3)(x4) en Related Symbolab blog posts Middle School Math Solutions – Equation Calculator Welcome to our new Getting Started math solutions series Over theFurther, we note that if g(x) = 1x2 then g′(x) = 2x So the integral Z 2x √ 1x2 dx is of the form Z f(g(x))g′(x)dx To perform the integration we used the substitution u = 1 x2 In the general case it will be appropriate to try substituting u = g(x) Then du = du dx dx = g′(x)dx Once the substitution was made the resulting integralX The Moonshot Factory X is a diverse group of inventors and entrepreneurs who build and launch technologies that aim to improve the lives of millions, even billions, of people Our goal 10x impact on the world's most intractable problems, not just 10% improvement

If X 1 2x 2 What Is The Value Of 8x 3 1 X 3 Quora

What Is The Value Of X 4 1 X 4 When X 1 X 2 Quora

F(x) = 4x^3 4x^2 x 1 f(1) = 4(1)^3 4(1)^2 (1) 1 = 441–1 = 0 So (x1) is a factor (4x^3 4x^2 x 1) ÷Explanation 4x −2x = 0 4x = 2x Apply the natural logarithm to both sides ln(4x) = ln(2x) Recalling that ln(ab) = bln(a) xln(4) = xln(2) So, x = 0 is obviously a solution as it will result in 0 = 0 Beyond that, there are no solutions as x cancels out and we're left withSince our derivation was symmetric in a and b, one of these roots can be used for a and the other for b, to find x4 x3 x2 x 1 = (x2 (1 2 √5 2)x 1)(x2 (1 2 − √5 2)x 1) If we want to factor further, use the quadratic formula on each of these quadratic factors to find the linear factors with Complex coefficients Answer link

Is X 3 4x 2 X 1 X 2 3 A Quadratic Equation

Free Math Answers Answers Within 24 Hours Step By Step Explanations

Solution to Problem 44 Use the difference of two squares to factor \( 16 x^4 81 = (4x^2 9)(4x^2 9)\) The term \( 4x^2 9 \) is a difference of two squares but we need to write \( 4x^2 9 \) as a difference of two squares using the imaginary unit \(i\) \( (4x^2 9)(4x^2 9) = (4x^2 9)(4x^2 (3i)^2) \) Factor again using theThen, f(x)g(x) = 4x 2 4x 1 = 1 Thus deg( f ⋅ g ) = 0 which is not greater than the degrees of f and g (which each had degree 1) Since the norm function is not defined for the zero element of the ring, we consider the degree of the polynomial f ( x ) = 0 to also be undefined so that it follows the rules of a norm in a Euclidean domainThen the inverse function f1 turns the banana back to the apple Example Using the formulas from above, we can start with x=4 f(4) = 2×43 = 11 We can then use the inverse on the 11 f1 (11) = (113)/2 = 4 And we magically get 4 back again!

Secure Media Collegeboard Org Digitalservices Pdf Ap Apcentral Ap15 Calculus Ab Q2 Pdf

Free Math Answers Answers Within 24 Hours Step By Step Explanations

Compute answers using Wolfram's breakthrough technology &Solution for X/x24/x2=1 equation X/X24/X2=1 We move all terms to the left X/X24/X2 (1)=0 Domain of the equation X!=0 X∈R We add all the numbers together, and all the variables X/X4/X1=0 Fractions to decimalsX 4 x 2 2 = 0 becomes y 2 y 2 = 0 We can factor this as (y2)(y1) = 0, which has solutions y = 2 and y = 1 Now, recall that we made the substitution y = x 2 Thus, we have x 2 = 2 or x 2 = 1 Thus, from x 2 = 2, we get x = ±i√2, and from x 2 = 1, we get x = ±1 Then, the solution is b

How To Do This Question X 3 1 2 Find Value Of 4x 3 2x 2 8x 7 Mathematics Topperlearning Com 4r5prjll

Ex 2 2 1 Find Value Of Polynomial 5x 4x2 3 At Ex 2 2

Solve `4x^28x4=0` `4(x^22x1)=0` factor out the common 4 `4(x1)^2=0` recognize `x^22x1` as a perfect square trinomialDivide x 1 − 2 4 x 2 − 1 by x 2 − 4 4 x 2 by multiplying x 1 − 2 4 x 2 − 1 by the reciprocal of x 2 − 4 4 x 2 Calculate x to the power of 1 and get x Calculate x to the power of 1 and get x Factor the expressions that are not already factored Factor the expressions that are not already factoredThe Algebra of Functions Like terms, functions may be combined by addition, subtraction, multiplication or division Example 1 Given f ( x ) = 2x 1 and g ( x ) = x2 2x – 1 find ( f g ) ( x

Www3 Nd Edu Apilking Math Work Old exams Exam3af15solutions Pdf

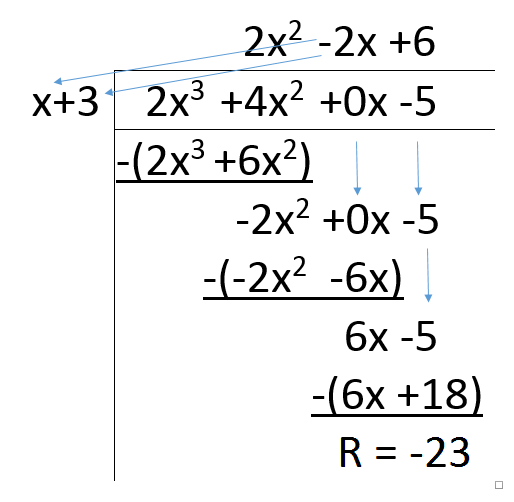
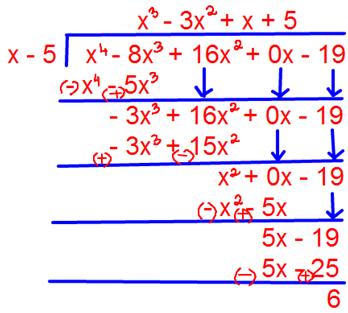
How Do I Use Long Division To Simplify 2x 3 4x 2 5 X 3 Socratic

13 x – 1 – (x – 1) 2 ax – a Solutionx – 1 – (x – 1) 2 ax – a By expanding the above we get, X – 1 – (x 2 1 – 2x) ax – a x – 1 – x 21 2x ax – a 2x – x 2 ax – 2 x – a Take out common in all terms, x(2 – x a) – 1(2 – x a) (2 – x a) (x – 1) Exercise 43 Factorise theIn mathematics, trigonometric substitution is the substitution of trigonometric functions for other expressions In calculus, trigonometric substitution is a technique for evaluating integralsMoreover, one may use the trigonometric identities to simplify certain integrals containing radical expressions Like other methods of integration by substitution, when evaluating a definite integral, it

1

Free Math Answers Answers Within 24 Hours Step By Step Explanations

If Y X 1 X Then X 4 X 3 4x 2 X 1 Becomes

X 1 X 1 2 Then Find 4x Square 4 X Square

Efisd Net Common Pages Displayfile Aspx Itemid

If The Number X 2 4x 1 And 5x 2 Are In Ap Then Find The Value Of X Arithmetic Progressions Maths Class 10

What Is The Value Of Math X 3 Dfrac 1 8x 3 Math If Math X 2 Dfrac 1 4x 2 8 Math Quora

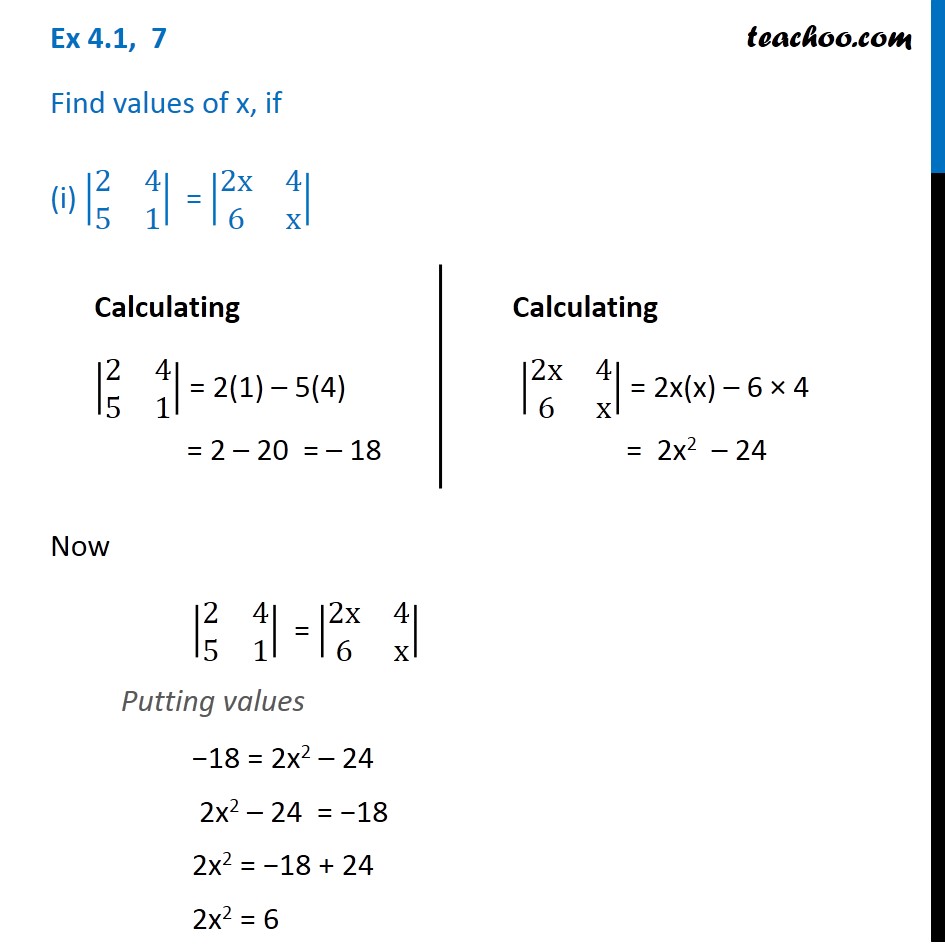
Ex 4 1 7 Find X If I 2 4 5 1 2x 4 6 X Determinants

What Is The Limit As X Approaches Infinity Of X 2 4 2x 4x 2 Socratic

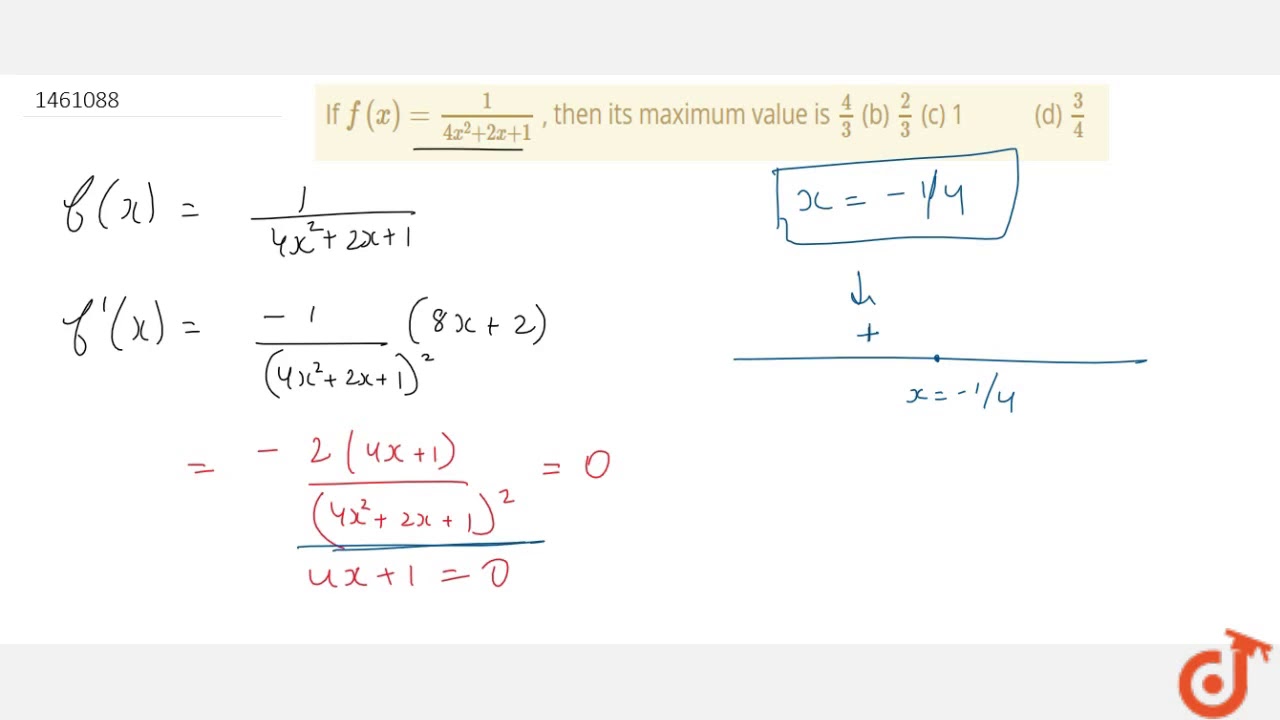
If F X 1 4x 2 2x 1 Then Its Maximum Value Is 4 3 B 2 3 C 1 D 3 4 Youtube

If F X E 2x 1 4x 1 2 Ln 1 X 2 For X 0 Then

Find The Value Of X For Which Numbers 5x 2 4x 1 And X 2 Are In A P Brainly In

1 2 4 8 Wikipedia

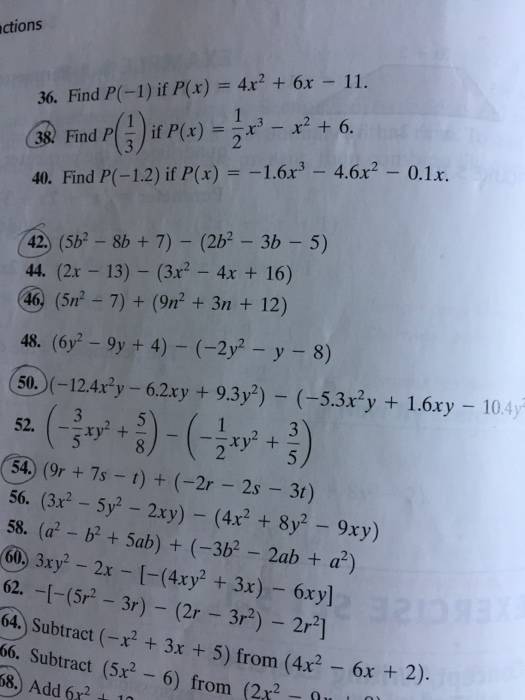
Find P 1 If P X 4x 2 6x 11 Find P 1 3 If Chegg Com

Ml Aggarwal Solutions For Class 10 Maths Chapter 6 Factorization Access Free Pdf

If Log 2 X2 4x 7 2 Then Find The Value Of X Brainly In

1

Ehsgiftedacademy Weebly Com Uploads 1 4 0 2 16 Honors Precalculus Summer Packet Answer Key 3 0 Pdf

What Is The Value Of 4x 3 2x 2 8x 7 If X 3 1 2 Quora

If 4x 4x 1 24 Then 2x X Equals What Polynomials Maths Class 9

Polynomial Functions

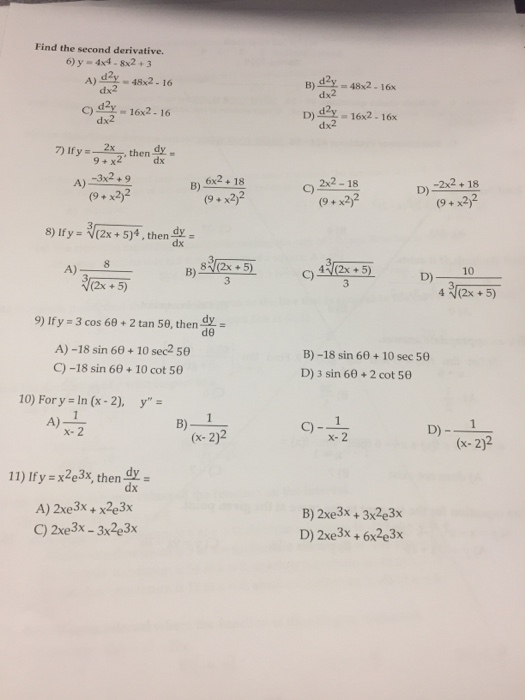
Find The Limit 1 Lim X 3 2 X 2 3 D 4 C 16 B Chegg Com

Find The Derivative Of A F X X 2 4 X 2 4 B Chegg Com

Solve For X X 1x 1 X 2x 2 4 2x 3x 2 X 1 2 2

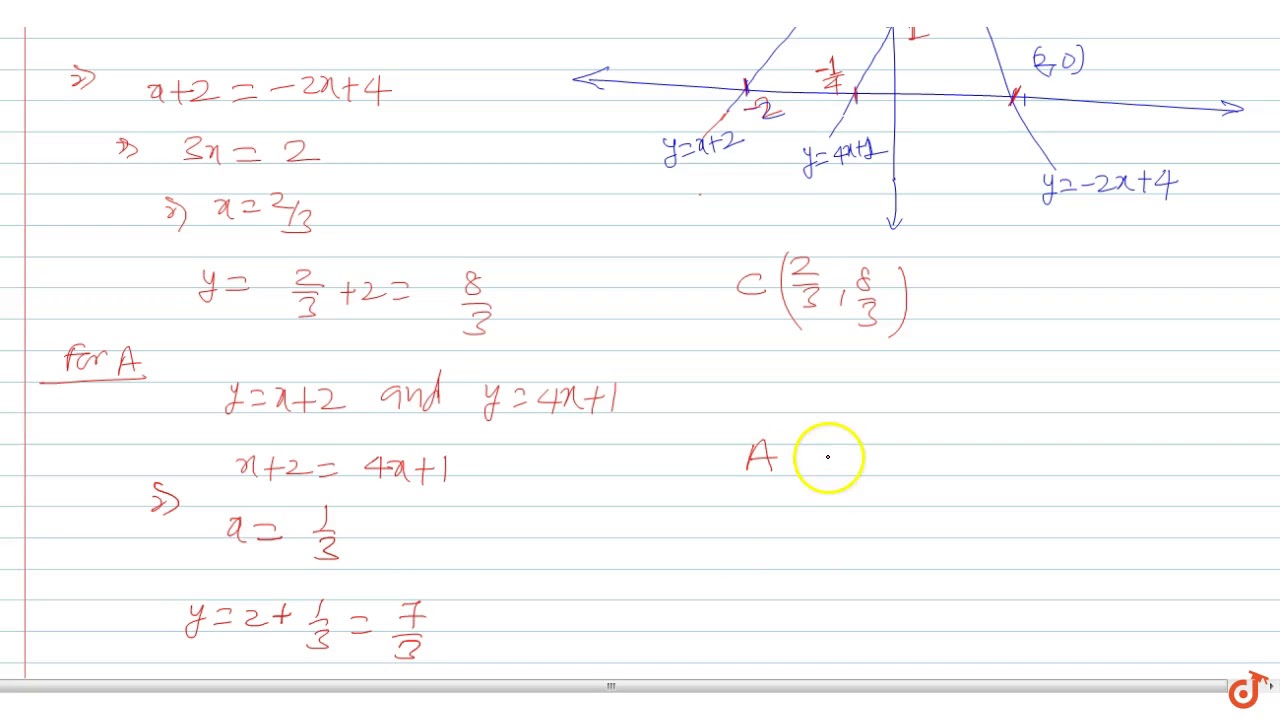
Systems Of Equations With Substitution Y 1 4x 100 Y 1 4x 1 Video Khan Academy

If P X X2 4x 3 Then Evaluate P 2 P 1 P 1 2 Studyrankersonline

What Is The Value Of 4x 3 2x 2 8x 7 If X 3 1 2 Quora

Step By Step Solutions

Ellipses And Hyperbolae

Limits By Factoring Video Khan Academy

If X 1 2 What Is The Value Of X 4 4x 3 4x 2 Quora

If X 1 X 1 2 Then Write The Value Of 4x 2 4 X 2 Maths Polynomials Meritnation Com

Http Www Mpsaz Org Rmhs Staff Lxcoleman Trig Test Practice Files Review Chapter 3 Notes Solutions Pdf

Www Deerfield K12 Wi Us Faculty Polzinr Cms Files Assignment Attach 330 Final review key Pdf

If 3 2x 1 4 X 1 36 Then Find The Value Of X

Www Math Utah Edu Wortman 1050 Text If Pdf

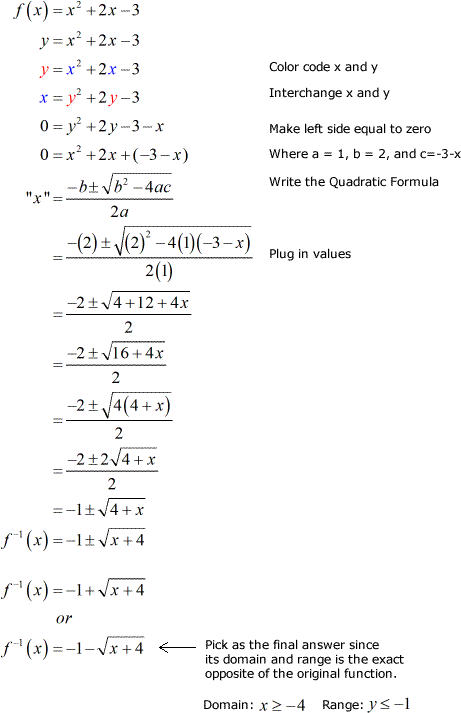
Inverse Of Quadratic Function Chilimath

Suppose U X Y 4x2 3y2 1 Calculate U X U Y 2 Evaluate These Partial Derivatives At Homeworklib

Solved If F X 1 X 2 4x 8 Then F X 1 Brainly In

2 2x 2 4 2x 1 Find The Value Of X Brainly In

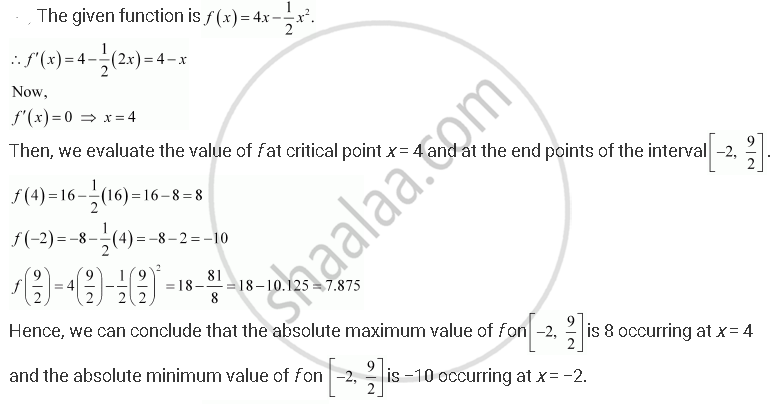
Find The Absolute Maximum Value And The Absolute Minimum Value Of The Following Functions In The Given Intervals F X 4x 1 X X 2 X In 2 9 2 Mathematics Shaalaa Com

Www Mpsaz Org Rmhs Staff Esritter Algebra2 Files Ch 1 2 Review Key Pdf

If X 2 1 4x 2 8 Find X 3 1 8x 3 Brainly In

Http Pages Stat Wisc Edu Ifischer Calculus Pdf

Which Of The Following Is A Quadratic Equation X2 2x 1 4 X 2 3 Studyrankersonline

Q Tbn And9gcq1flnomd Pujumzkbsjbtybsev2vjutm3qqtulh97pbh3cdumb Usqp Cau

Linear Equations 4 Video Khan Academy

Math Scene Equations Iii Lesson 2 Quadratic Equations

Solve Solve Inequalities With Step By Step Math Problem Solver

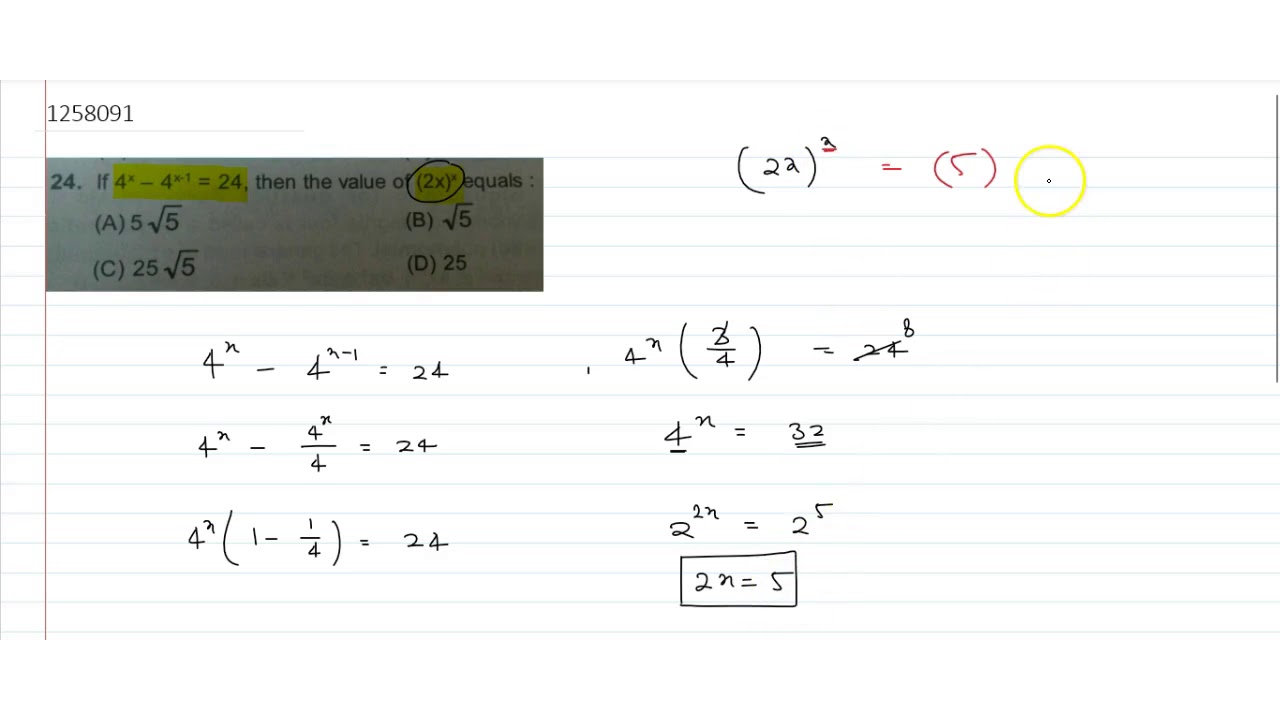
If 4 X 4 X 1 24 Then The Value Of 2x X Equals Youtube

Www Southhadleyschools Org Cms Lib Ma Centricity Domain 11 5 4 Pdf

Solve For X 4x 3 2x 1 10 2x 1 4x 3 3 X 1 2 3 4

Free Math Answers Answers Within 24 Hours Step By Step Explanations

Chapter 1

Irreducible Polynomial Wikipedia

Example 25 Let F X 4x 2 12x 15 Show That F Is Invertible

Wolfram Alpha Examples Step By Step Solutions

Differentiate Each Function 1 Y 3 2x 2 2 Y Chegg Com

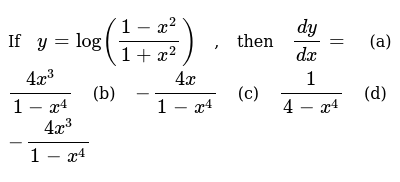
X 1 X 1 2 Then Find 4x Square 4 X Square

Ftre 16 If X 1 X 4 Then X 5 1 X 5 Youtube

If X 1 X 5 Then Is X 2 1 X 2 Equal To Quora

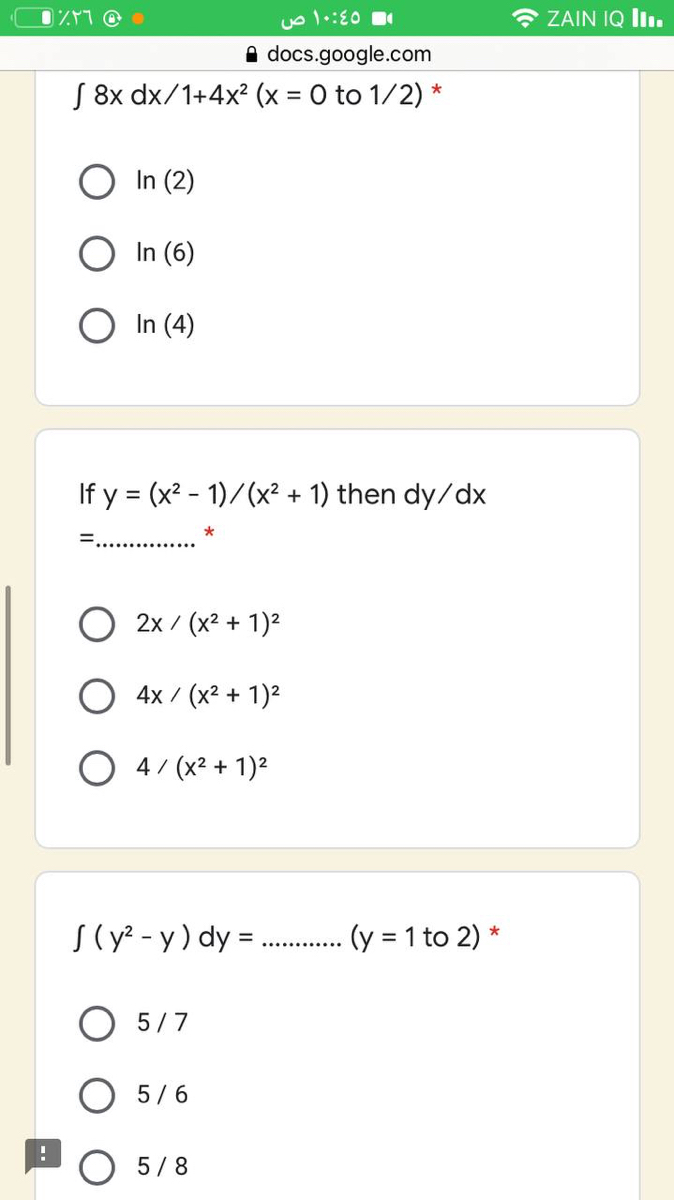
Answered S 8x Dx 1 4x X 0 To 1 2 O In 2 Bartleby

1 1 X24x4 See How To Solve It At Qanda

Math Scene Equations Iii Lesson 2 Quadratic Equations

If X 1 X 1 2 Then Write The Value Of 4x 2 4 X2

Www Jmap Org Worksheets A Sse A 2 Factoringpolynomials4 Pdf

Q5 If X 1 X 1 2 Then Write The Value Of 4x 2 4 X 2

On Dividing The Polynomial P X 9x4 4x2 4 By The Polynomial G X 3x2 X 1 The Remainder Is Ax B Find A And B Mathematics Topperlearning Com Ab5z94lss

Evaluate The Following X 4 4x 3 6x 2 4x 9 When X 1 I 2

If X 1 2 What Is The Value Of X 4 4x 3 4x 2 Quora